Prospect Theory: Trading Prospect Capital
In my last blog post, I referenced behavioral economics and tried to explain why field managers and front offices make certain decisions. There was one behavioral economics theory – the most commonly known one – that I left out, Prospect Theory. In traditional loss aversion stories – from expected utility theory – the individual only cares about their final wealth position. In this traditional model, an individual will maximize their utility subject to some constraint. For baseball, this would be a General Manager trying to maximize their wins subject to not only their budget constraint but also the roster constraint, both the 26-man active roster and the 40-man roster. For a manager, that could be reflected in maximizing their win probability subject to the inning along with what pitchers and hitters are available out of the bullpen and off the bench. The focus is on the end goal. In expected utility theory, that would be the final wealth levels and in our baseball example, it would be the amount of wins and win probability.
Prospect Theory takes a different look, however. This theory was developed by Daniel Kahneman and Amos Tversky in 1979, which simply states that people use a value function relative to a reference point (anchoring) and use decision weights (perceived likelihood of the event) to evaluate decisions.
As an example, assume that the initial wealth of an individual is $1,000 and they have a lottery A such that they lose $500 with probability 0.5 and win $500 with probability 0.5, or simply A = {(-500, 0.5), (500, 0.5)}. Let’s also imagine a world where instead of having $1,000 to start, they have $800 with a lottery B that pays {(-300, 0.5), (700, 0.5)}. The expected wealth in the first scenario is $1,000 + (-500*0.5 + 500*0.5) = $1,000 and the second scenario is $900 + (-300*0.5 + 700*0.5) = $1,000. In this situation, the utility maximizer would be indifferent to the choices as they both end at $1,000. But what Prospect Theory states is that the person would prefer B to A (the loss is not as much):

This value function is steeper for losses than gains and is the steepest at the reference point. This is because people have a prior probability that they assign to the event as they do not know the underlying probability distribution. In the paper, Kahneman and Tversky show a similar image to the one below in regards to what is called the decision weight:
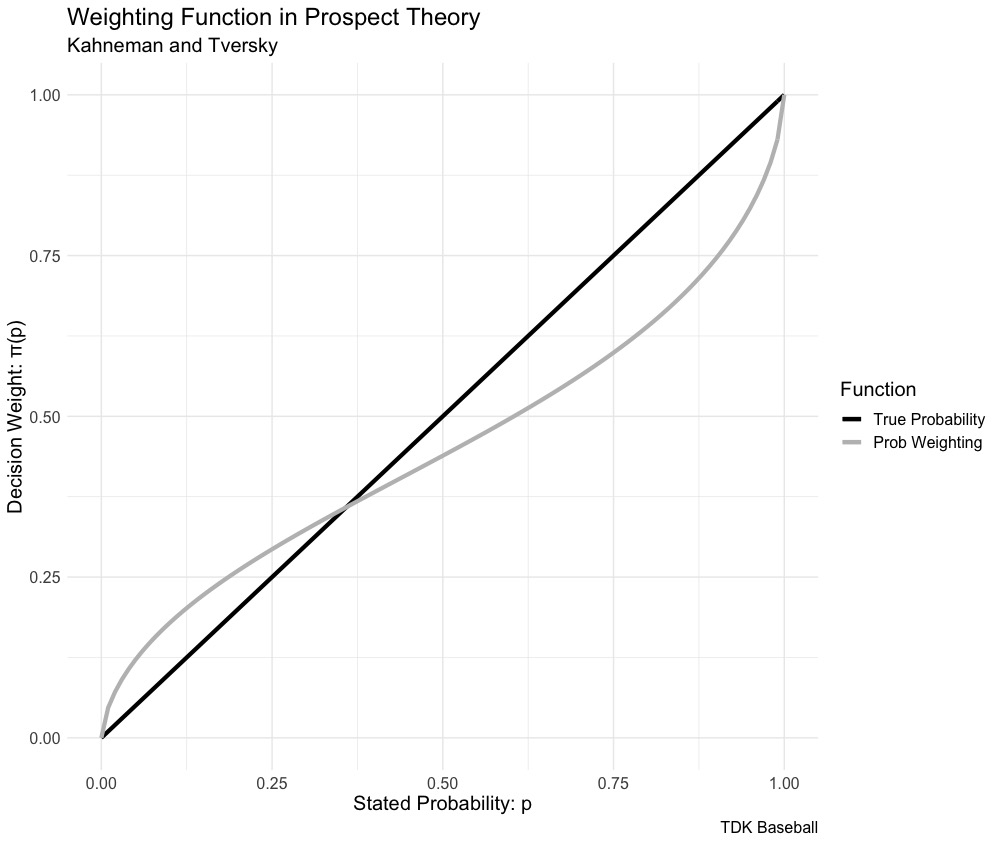
Individuals tend to overweight low probability events (think of playing the lottery) and underweight high probability events (I don’t have a good example for this, but perhaps a team up three in the top of ninth who decides to use their closer despite a win probability of 97.89 percent). In prospect theory, the magnitude is important – in Misbehaving by Richard Thaler, the estimate is losses hurt twice as much as gains – and individuals are not well calibrated to the true probability.
Risk aversion can be seen all across baseball. Instead of going after a top of the rotation arm in Lance Lynn or Mike Clevinger, or even a mid rotation starter such as Joe Musgrove, the Braves turned towards Tommy Milone. The Braves didn’t want to trade from their prospect pool, one that ranks 17th in baseball per FanGraphs. While AJ Preller was slinging trades, he only traded away one top prospect in Taylor Trammell, preferring to keep other top prospects in the system instead. This can be explained through Prospect Theory.
Zach Kram of The Ringer looked at trades of top prospects and found, “More than half of all top-50 prospects in this sample accumulated less than 6 WAR in their first six seasons, and even top-10 prospects—the best of the best—failed to record even 1 WAR per season more than a third of the time.” There is asymmetric information, the team trading the prospect knows more about the player than the team acquiring, but there is risk in trading abd acquiring top prospects, and this is where Prospect Theory comes into play.
A General Manager doesn’t want the trade to backfire and cost them down the line (perhaps signaling they are not discounting the future enough) and overstating the probability that the prospects traded will blossom into top players and potentially understating the probability that the player acquired works out, but it’s also in terms of the reference point, in this case the player evaluation. Because top prospects are even likely to bust, a General Manager should be willing to trade those prospects, they’re just hesitant because of the overweighting in probability that the trade will backfire and the fact that the loss of a prospect turning into a star will hurt more than the improvements in the current year. The team acquiring the prospects is willing to make the trade because of the higher talent potential that will pay off for in the long run. There is perceived less risk in acquiring top prospects (reference points), but risk nonetheless. A General Manager is going to be loss averse given the organization structure of the sport (my post from last week), but as Kiley McDaniel wrote, being aggressive is the inefficiency. A team who is willing to be more calibrated to the true probabilities with a proper discount rate, will see better returns, at least in the short run, and General Managers are evaluated on the short run.
***
Another problem in current prospect evaluation is teams valuing players the same way. Despite the lessons two decades ago in Moneyball, zag when others zig, everybody is zigging. Thaler, in Misbehaving, summarized findings by Benjamin Graham in Intelligent Investor, writing: “Cheap stocks were unpopular or out of favor, while expensive stocks were fashionable. By being a contrarian, Graham argued, you could beat the market, although not all the time.” Zag while others zig, the story of Moneyball, just written in 1949 and revised in 1973 (later updated by others).
The Moneyball theory continues: “Graham noted that his strategy of buying the cheapest members of the Dow Jones Industrials would not have worked over an earlier period, 1917-1933, and he cautioned ‘Undervaluations caused by neglect or prejudice may persist for an inconveniently long time, and the same applies to inflated prices caused by overenthusiasm or artificial stimulants.'” The concepts are old; you cannot expect to profit by following the market, you have to zag. In terms of prospects, given the reliance on statistical models, evaluations will be similar for higher level prospects. The zag is then the scouting, and targeting younger and raw prospects. With less data, a scouting presence matters and is important. To really build a farm for rebuilding, and to potentially find the inefficiency, is to acquire prospects before their breakout because once they do, a team won’t be able to poach them.
0 Comments on “Prospect Theory: Trading Prospect Capital”